how to draw a factor tree for 64

Factor Tree

A factor tree is created to find the prime factors of a number. It is created in the form of a tree in which the given number is split into branches that represent all the factors of the number. Let us learn more about finding the factors of a number using the factor tree method.

What is a Factor Tree?

A factor tree is a method of factorizing a number that is similar to the way in which the branches of a tree are split. Every branch of the factor tree is split into factors and once the factors cannot be factorized further, the branches come to an end, the final factors are circled and are considered to be the prime factors of the given number.

How to Draw a Factor Tree?

A factor tree can be drawn by factorizing a number until we reach its prime factors. These factors are split and written in the form of a tree. Let us see how to draw the factor tree of 24.

Factor Tree of 24

The prime factorization of a number can be done by using the factor tree method. This is done by drawing the branches of the given number and writing the factors at the end of each branch. Once we reach that number which cannot be split any further, we stop and the factor tree is complete because all its prime factors are listed. At this stage, all the prime numbers that were circled are listed as the prime factors of the given number. Let us understand this by finding the factors of 24 using the factor tree method.

The factors of 24 can be calculated using the following steps. Observe the following figure to see the factor tree of 24.

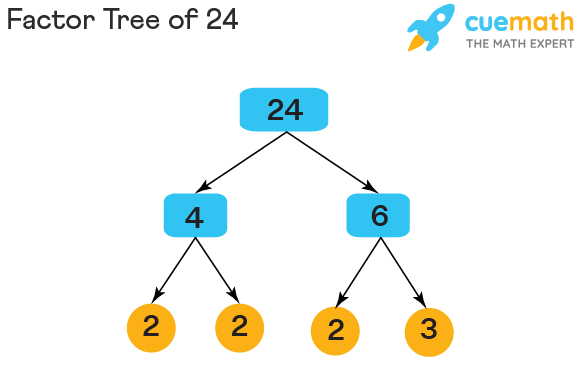
• Step 1: Find two factors of 24. We get 4 and 6.
• Step 2: Observe these factors to see if they are prime or not.
• Step 3: Since both 4 and 6 are composite numbers, they can be further split into more factors. Hence, we repeat the process of factorizing them and splitting them into branches until we reach the prime numbers.
• Step 4: Here, 4 can be further split into 2 and 2. Similarly, 6 can be further split into 2 and 3. At this stage, we reach the prime numbers, 2 and 3. We circle them since we know that they cannot be factorized further. This is the end of the factor tree.
• Step 5: Finally, we list all the circled factors as the prime factors of 24. This shows that the prime factors of 24 = 2 × 2 × 2 × 3

Factor Tree of 36

The factor tree of 36 can be drawn using the following steps.

• Step 1: We start factorizing 36 and we split it into 2 and 18.
• Step 2: Since 2 is a prime number, we cannot factorize it further, so, we circle it and we will come back to it later. However, 18 can be split further into 2 and 9.
• Step 3: We know that 2 is a prime number so we circle this 2 since it cannot be split further. But, we can factorize 9 into 3 and 3.
• Step 4: After factorizing 9 into 3 and 3, we finally reach the stage when all the factors are prime numbers. Hence, we stop and the factor tree of 36 is complete.
• Step 5: Now, we list all the circled factors as the prime factors of 36. This shows that the prime factors of 36 = 2 × 2 × 3 × 3

Factor Tree of 48

The factors of 48 can be easily listed by drawing the factor tree of 48. Let us factorize 48 using the factor tree method.

• Step 1: We start factorizing 48 and we split it into 6 and 8.
• Step 2: It can be seen that 6 is split into 3 and 2; whereas, 8 can be split into 4 and 2.
• Step 3: While we circle the prime numbers till this step, we still need to factorize 4 into 2 and 2.
• Step 4: At this step, we have reached all the prime factors of 48 which cannot be factorized any further. This completes the factor tree and the prime factors of 48 can be listed.
• Step 5: This shows that the prime factors of 48 = 3 × 2 × 2 × 2 × 2

☛ Related Articles

• Factors and Multiples
• Factors and Multiples Worksheets
• Factors of 1
• Factorization of Algebraic Expressions
• Prime Factorization Calculator

Factor Tree Examples

1. Example 1: Fill in the missing numbers in the factor tree of 99.

   

   Solution: 99 can be split into 3 and 33. Then, 3 can be circled since it is a prime number and we further split 33 into 3 and 11. Therefore,

   a.) The number in the first missing blue box is 33.

   b.) The number in the second missing yellow circle is 3.

2. Example 2: Write the factors of 16 using a factor tree.

   Solution: The factors of 16 can be calculated with the help of the factor tree.

   

   This shows that the prime factors of 16 = 2 × 2 × 2 × 2

3. Example 3: State true or false.

   a.) The factors of 24 are: 2 × 2 × 2 × 3

   b.) A factor tree can be drawn by splitting the factors of a number until we reach its prime factors.

   c.) A factor tree does not find the prime factors of a number.

   Solution:

   a.) True, the factors of 24 are: 2 × 2 × 2 × 3

   b.) True, a factor tree can be drawn by splitting the factors of a number until we reach its prime factors.

   c.) False, a factor tree finds the prime factors of a number.

View Answer >

go to slidego to slidego to slide

Ready to see the world through math's eyes?

Math is a life skill. Help your child perfect it through real-world application.

Book a Free Trial Class

Practice Questions on Factor Tree

Check Answer >

go to slidego to slide

FAQs on Factor Tree

What is a Factor Tree?

A factor tree is created to find the prime factors of a number. It is a method of factorizing a number in which the factors are split and written in such a way that it looks like the branches of a tree.

How Does a Factor Tree Work?

A factor tree works according to the usual way of factorizing a number. The only distinct feature is that the factors are split and written in a way that it appears like a tree in which the branches are split. The following steps show the way in which a factor tree works. Let us understand this by factorizing the number 28.

• Step 1: We start factorizing 28 and we split it into 2 and 14.
• Step 2: Now, 2 is a prime number, so we will circle it and move ahead to factorize 14 further.
• Step 3: 14 can be factorized into 2 and 7. Since 2 and 7 are prime numbers, we stop here and circle 2 and 7.
• Step 4: At this step, we have reached all the prime factors of 28 which cannot be factorized any further. This completes the factor tree and we list all the circled prime numbers as the prime factors of 28. This is represented as 28 = 2 × 2 × 7.

What is the Factor Tree of Hundred?

The factor tree of 100 can be drawn easily using the following steps.

• Step 1: We start factorizing 100 and we split it into 2 and 50.
• Step 2: Now, 2 is a prime number, so we will circle it and move ahead to factorize 50.
• Step 3: 50 can be factorized into 2 and 25.
• Step 4: After circling 2 at this step, we will factorize 25 into 5 and 5.
• Step 5: At this step, we reach the stage where the factors can no more be factorized. This means we can list the circled factors as the prime factors of 100 as 100 = 2 × 2 × 5 × 5.

How to Find Prime factors Using Factor Tree?

The prime factors of a number can be listed using a factor tree. We start splitting the number by listing its factors until the factors cannot be split anymore. These factors are split and written like the branches of a tree. Once we start splitting, we circle the prime numbers and move ahead to factorize the composite numbers further. After we reach the stage where the factors cannot be split any further, we come back to the circled prime factors at each step and list these numbers as the prime factors of the given number.

How to Find LCM Using Factor Tree?

The Least Common Multiple (LCM) of two numbers is the least number which is a common multiple for both the numbers. We can find the LCM of numbers using a factor tree. For example, let us find the LCM of 6 and 8 using a factor tree.

• Step 1: We will start factorizing 6 and split it into 2 and 3.
• Step 2: Now, since 2 and 3 are prime numbers, we will circle them as the prime factors of 6.
• Step 3: Then, we will factorize 8 into 2 and 4. We will circle 2 since it is a prime number and we will factorize 4 further into 2 and 2.
• Step 4: So, the prime factors of 8 are 2 × 2 × 2.
• Step 5: Now, we have the prime factorization of 6 and 8 and this can be expressed as, 6 = 2 × 3 and 8 = 2³. We will observe these factors to move further.
• Step 6: We know that LCM is the product of the largest multiple of every prime number that is present on at least one list. For example, we have a 2 and a 3. So, we will choose the largest multiples of 2 and 3 in this list and find their product. The largest multiple of 2 here is 2³ and the largest multiple of 3, in this case, is 3. This means, the LCM of 6 and 8 = 2³ × 3 = 24

How to Find GCF Using Factor Tree?

The Greatest Common Factor (GCF) of two numbers can be calculated using a factor tree. For example, let us find the GCF of 20 and 30 with the help of a factor tree.

• Step 1: We will start factorizing 20 and split it into 2 and 10.
• Step 2: Since 2 is a prime number, we will circle it and we will factorize 10 into 2 and 5. After circling 2 and 5, we can list the prime factors of 20 as, 20 = 2 × 2 × 5.
• Step 3: Then, we will factorize 30 into 5 and 6. Since 5 is a prime number, we will circle it and we will further factorize 6 into 2 and 3. After circling 2 and 3 we get the prime factors of 30 as, 30 = 2 × 3 × 5.
• Step 4: Now that we have the prime factors of both the given numbers we can list them as, 20 = 2 × 2 × 5 and 30 = 2 × 3 × 5.
• Step 5: We know that GCF is the product of the prime factors that are common to both factorizations. This means that in both factorizations, we have 2 and 5 which are the common factors and so the GCF of 20 and 30 will be the product of 2 and 5. This means, 2 × 5 = 10. Therefore, the GCF of 20 and 30 is 10.

What is the Factor Tree for 144?

The factor tree for 144 can be drawn using the following steps.

• Step 1: Find two factors of 144. We get 2 and 72.
• Step 2: Observe these factors to see if they are prime numbers or not. Since 2 is a prime number we will circle it and come back to it later.
• Step 3: We will further factorize 72 into 2 and 36. We will again circle 2 at this step since 2 is a prime number and move on to factorize 36. So, 36 can be factorized as 6 and 6. Since 6 is a composite number, it can be further split into 2 and 3. We will circle 2 and 3 since they are prime numbers. This is where the factor tree ends.
• Step 4: Now that we cannot factorize the factors any further, we will list all the circled factors which are the prime factors of 144. This means, 144 = 2 × 2 × 2 × 3 × 2 × 3.

What is the Factor Tree of 27?

In order to create a factor tree of 27, we use the following steps.

• Step 1: First, we will factorize 27 and split it into 3 and 9.
• Step 2: Since 3 is a prime number, we will circle it and come back to it later. Then, we will factorize 9 into 3 and 3. Since 3 is a prime number we will circle both the 3s and complete the factor tree.
• Step 3: At this step, we reach the stage where the factors cannot be factorized any further. This means we can list the circled factors as the prime factors of 27 as 27 = 3 × 3 × 3.

jordanshorme.blogspot.com

Source: https://www.cuemath.com/numbers/factor-tree/

Share this post